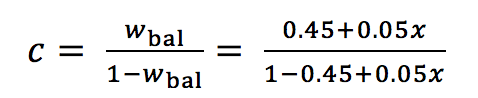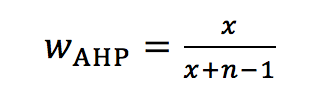As part of my current work about AHP scales, here an important finding for the balanced scale:
Salo and Hamalainen [1] pointed out that the integers from 1 to 9 yield local weights, which are not equally dispersed. Based on this observation, they proposed a balanced scale, where local weights are evenly dispersed over the weight range [0.1, 0.9]. They state that for a given set of priority vectors the corresponding ratios can be computed from the inverse relationship
r = w / (1 – w) (1a)
The priorities 0.1, 0.15, 0.2, … 0.8, 0.9 lead, for example, to the scale 1, 1.22, 1.5, 1.86, 2.33, 3.00, 4.00, 5.67 and 9.00. This scale can be computed by
wbal = 0.45 + 0.05 x (1b)
with x = 1 … 9 and
c ( resp. 1/c) are the entry values in the decision matrix, and x the pairwise comparison judgment on the scale 1 to 9.
In fact, eq. 1a or its inverse are the special case for one selected pairwise comparison of two criteria. If we take into account the complete n x n decision matrix for n criteria, the resulting weights for one criterion, judged as x-times more important than all others, can be calculated as:
Eq. 2 simplifies to eq. 1a for n=2.
With eq. 2 we can formulate the general case for the balanced scale, resulting in evenly dispersed weights for n criteria and a judgment x with x from 1 to M:
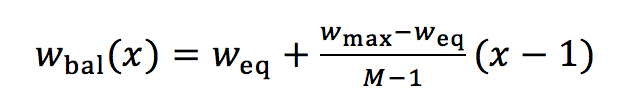 (3)
(3)
with
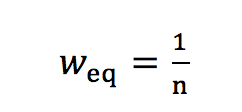 (3a)
(3a)
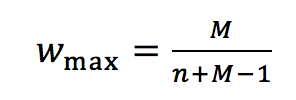 (3b)
(3b)
![]() (3c)
(3c)
We get the general balanced scale (balanced-n) as
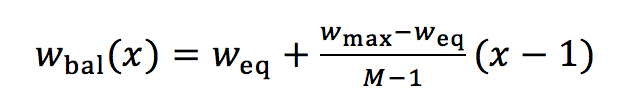 (4)
(4)
With n=2 and M=9 it represents the classical balanced scale as given in eq. 1b and 1c. Fig. 1 shows the weights as a function of judgements derived from a case with 7 criteria using the fundamental AHP, balanced and general balanced (bal-n) scale. It can be seen that, for example, a single judgement “5 – strong more important” yields to a weight of 45% on the AHP scale, 28% on the balanced scale and 37% on the balanced-n scale.
Figure 1. Weights as function of judgment for the AHP scale, the balanced scale and the corrected balanced scale for 7 decision criteria.
A “strong” criterion is underweighted using the classical balanced scale, and overweighted using the standard AHP scale, compared to the general balanced-n scale. Weights of the balanced-n scale are distributed evenly over the judgment range, and only for n = 2 the original proposed balanced scale yields evenly distributed weights.
You can download my complete working paper “Comparison of Judgment Scales of the Analytical Hierarchy Process – A New Approach“, submitted for publication.
References
[4] Salo, A.,Hämäläinen, R., On the measurement of preferences in the analytic hierarchy process, Journal of multi-critria decision analysis,Vol. 6, 309 – 319, (1997).